
By the time students reach middle school, they may have already internalized a belief that they are either “good” or “bad” at math. Reaching the students who have decided that math is boring and incomprehensible can be an uphill battle for teachers, especially if those students start the year with considerable knowledge gaps.
One way to circumvent math anxiety and re-engage the students who mentally check out when they hear the word “math,” is to make learning an active experience instead of relying on a passive transfer of information.
Active, experiential learning is at the core of ST MathⓇ. Each concept scaffolds visual models and gradually introduces symbolic representations and vocabulary, which means that at the beginning, the puzzles don’t look like textbook math at all.
Students gain hands-on experience by manipulating visual models and seeing how the results of their choices play out in real time. When they reach the harder levels where equations and formulas come into play, students already have the confidence and intrinsic motivation to keep on problem solving.
Keep reading to see an ST Math game in action!
Parabola Balloons is an eighth grade game that begins the “Graphing Parabolas” module. In an earlier objective, students learn how to graph linear equations with a similar game called Linear Balloons, so by the time they start on parabolas they already have context for what’s going on.
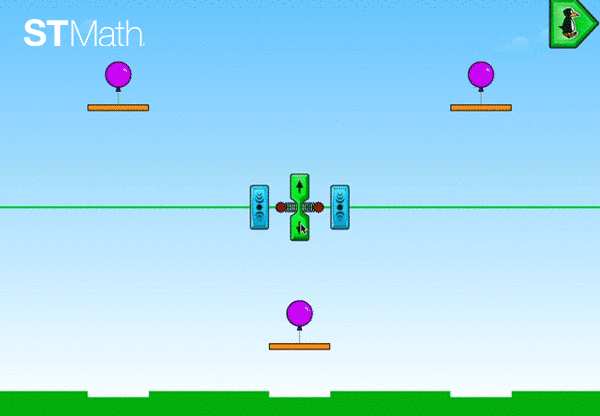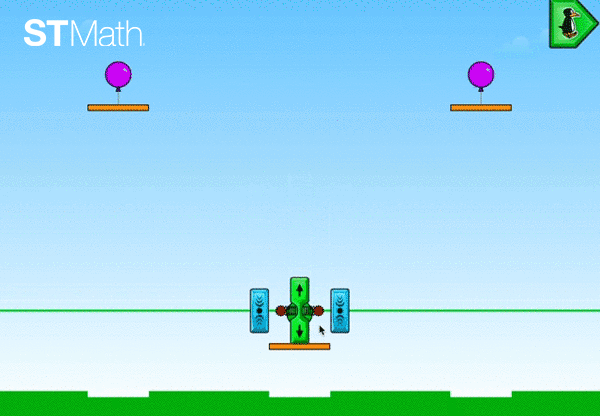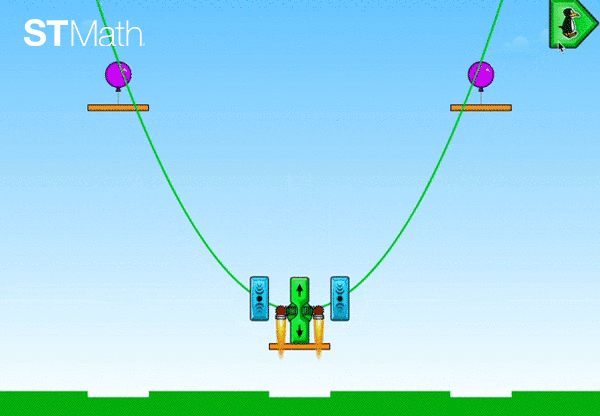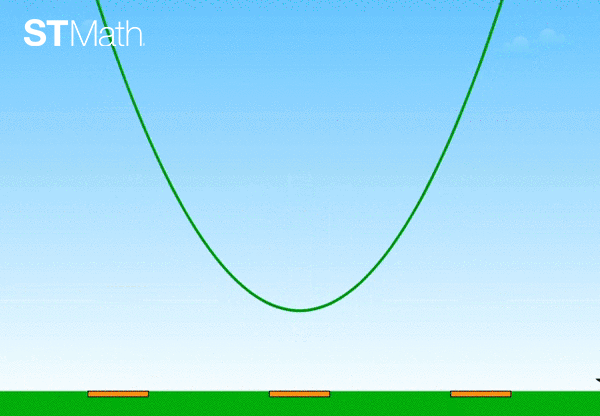
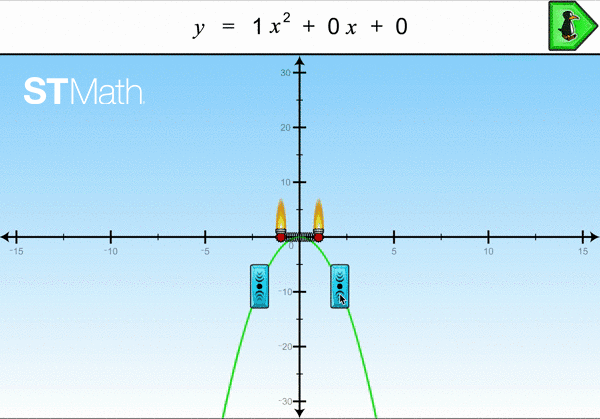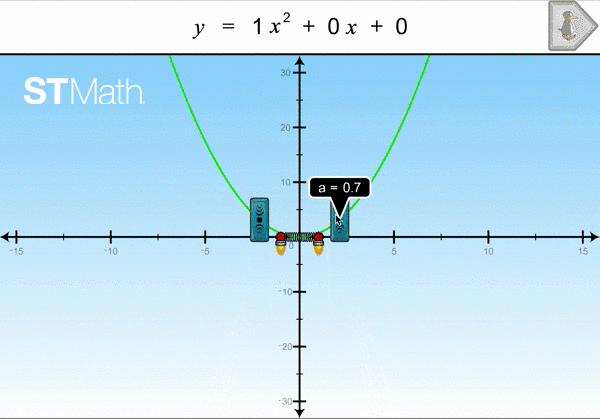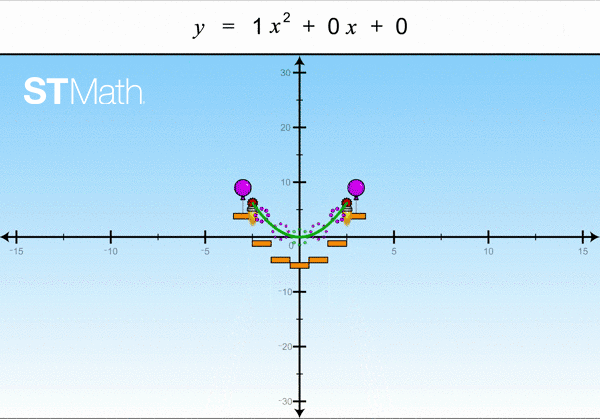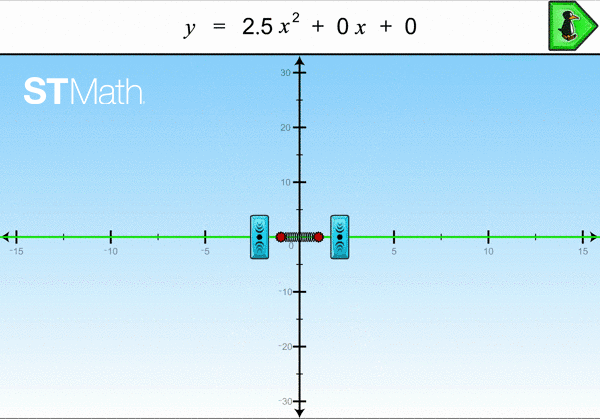
The first level of Parabola Balloons introduces a simple goal: pop the balloons and get JiJi to cross. The students manipulate the a-value of a quadratic equation to line it up with balloons and press “play” when they’re ready:
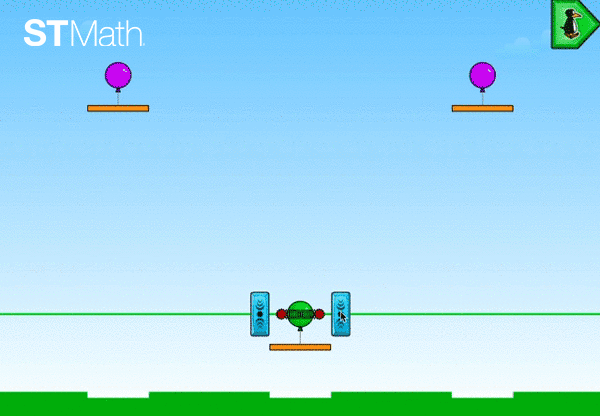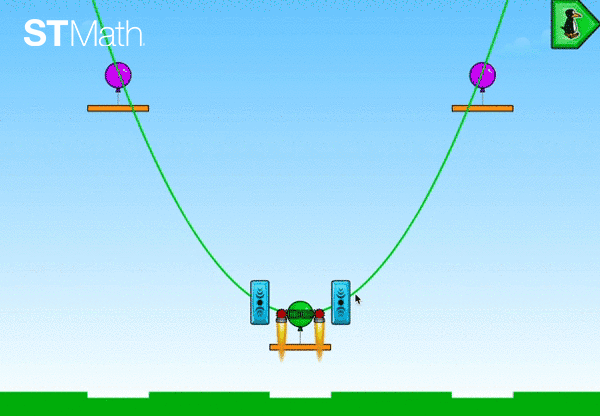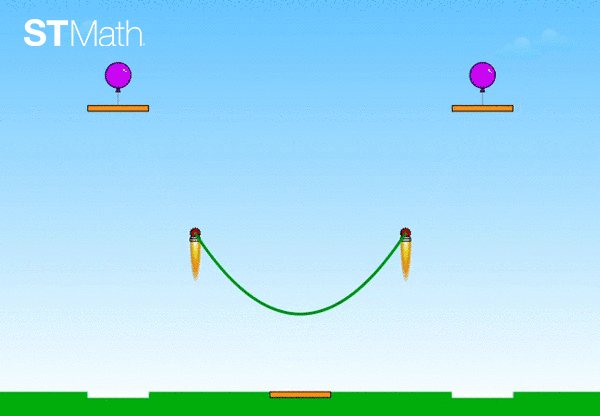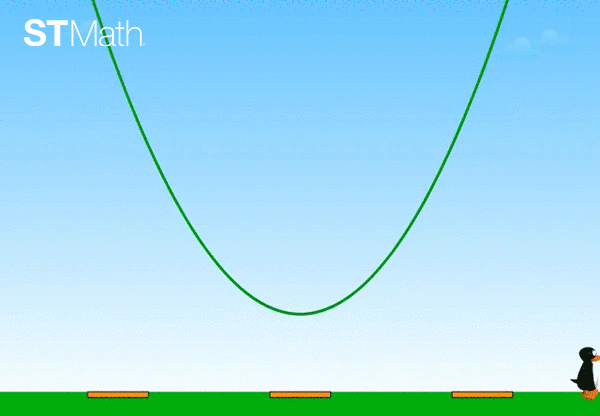
The animated, informative feedback that instantly follows each answer motivates students to keep going, whether their answer was correct or not.
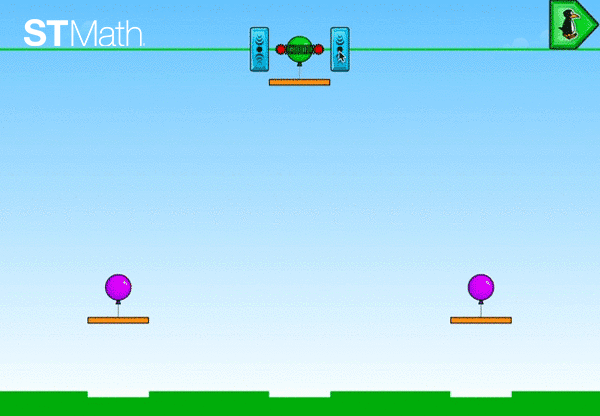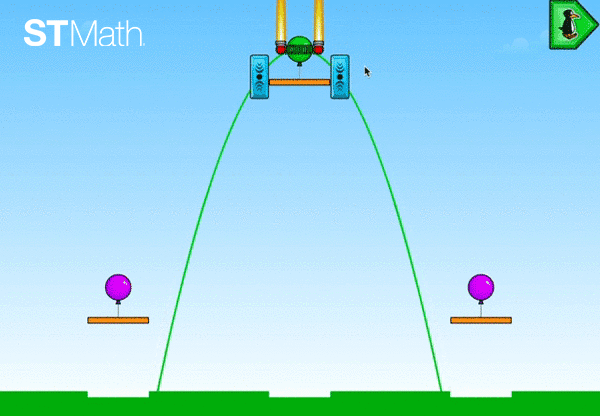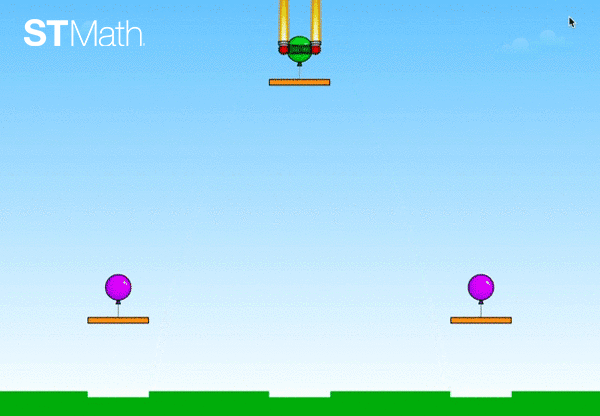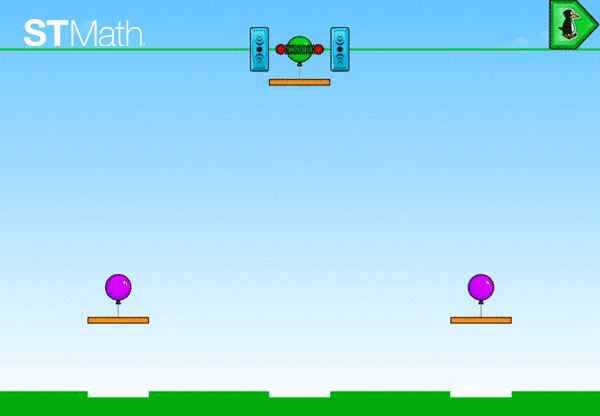
In cases where the student chooses incorrectly (like in the animation above), they don’t see a red X, a huge “Incorrect,” a hint or even a pop-up tutorial video. Students watch the animation, observe why their answer was incorrect, and they try again. ST Math encourages the same kind of perseverance kids have with video games: if you miss a jump in Super Mario Bros, you adjust your approach and keep trying.
Just like with any game, ST Math progresses in difficulty with each level, but because each element appears one at a time, the program challenges students without overwhelming them.
Further along in level one, students have to manipulate both the a-values and the y-intercept to create a parabola that intersects the balloons. The visual model allows students to make connections between the two parts of a quadratic equation through hands-on experience.
While students work in these initial, visual only models, teachers have the opportunity to step in and slowly introduce vocabulary, and ask students to explain what’s happening on the screen. Even when students whiz through the levels, asking them to slow down and explain their thinking facilitates academic discourse that can deepen their engagement. A teacher can ask:
Jumping a few steps ahead, this game shows the same exact mechanics as the previous one, but places them in context, complete with an equation and graph:
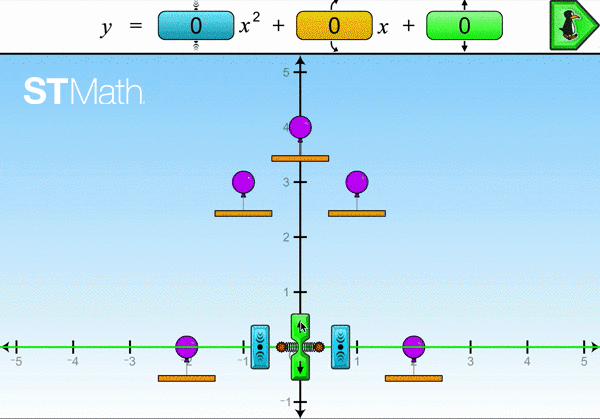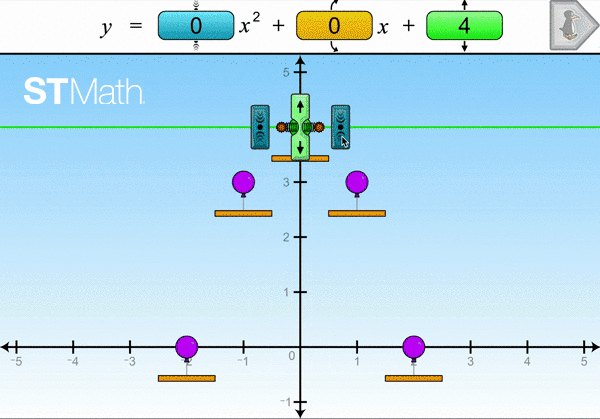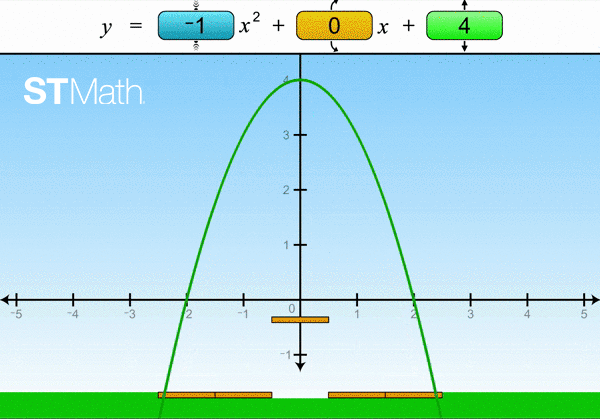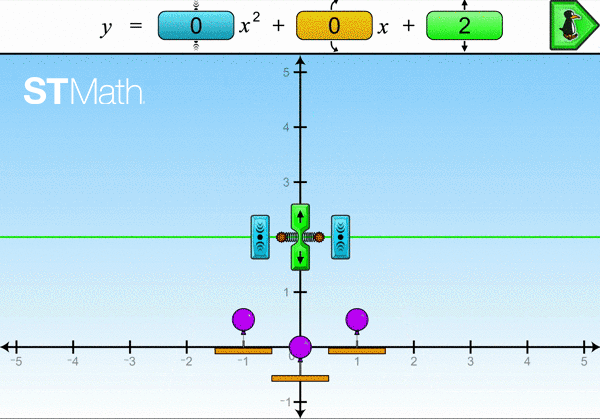
Notice how the definitions for y-intercept and a-values pop up whenever students manipulate each aspect of the graph, and how the colors correspond to the equation. These small details help students draw connections between the visuals and their representative symbols, all while keeping their focus.
In the second level of this game, the balloons are gone and students have to graph the equation they see without any hints -- essentially the same kind of question they’d see on an assessment. But even though balloons are no longer guiding the student to the right answer, the animated feedback still makes the same visual connections as the earlier levels.
If you enjoyed this glimpse into ST Math, you can try other sample games on our website, or find out more about ST Math for middle school. Check out the resources below to learn more about the science behind ST Math:

Ieva Galinyte is an associate product manager at MIND Research Institute. She enjoys writing about and keeping up with the latest trends in math education.
Comment