
In 1963, mathematician Stanislaw Ulam was doodling during a lecture. He was writing out positive integers (positive numbers counting by 1) in a grid where the number 1 was in the middle and the other numbers spiraled outward.
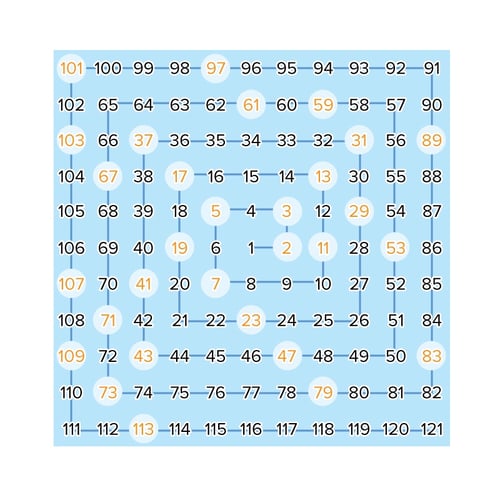
Once Ulam had completed his spiral, he decided to circle all of the prime numbers and discovered an unusual pattern. All of the prime numbers lined up in diagonals!
In order to make sure it wasn’t just coincidence, Ulam expanded his spiral to include more numbers and found that a larger grid made the pattern stand out even more.
The figure below is a 200 x 200 spiral where each black dot represents a prime number—you can clearly see the black dots creating diagonals in every direction. There are even diagonals of blank spaces where prime numbers are notably missing.
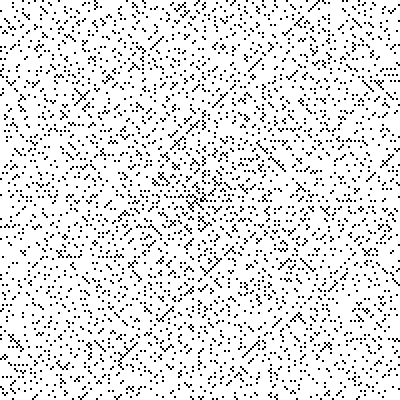
No one has yet figured out why prime numbers line up this way, but mathematicians agree that the patterns are significant (not to mention cool!).
Ulam discovered the connections between prime numbers, but there are many other patterns to find!
This activity is adaptable for grades K-8 and above—make it as complex or as simple as you like. Explore the number spiral by coloring, circling, or drawing to make connections between the numbers.
If you want a few suggestions to get started, check out our facilitation guide here.
Make sure to share your number spiral discoveries with us on social media and good luck exploring!
This activity is inspired by Megan Schmidt from her blog on Number Loving Beagle.

Ieva Galinyte is an associate product manager at MIND Research Institute. She enjoys writing about and keeping up with the latest trends in math education.
Comment