
It may seem that there is little overlap between math and art, but the merging of the two creates a synergy that enhances each subject, giving more meaning to the art and greater context to the math concepts being taught - creating a truly rich learning experience.
Any opportunity students have to create is a moment of empowerment. Math goes from being frustrating and mysterious to relatable and tangible when explored through hands-on art activities. Not only does this change students’ understanding and perception of math (go away, math anxiety!), experiences like this change the way students think of themselves as math learners.
Throughout history, people have used pattern art to exhibit and explore mathematical understanding. To help this connection come alive for your students, square paper tiles are great visual tools. (And easily accessible in the form of post-it notes or other square papers!) They are tangible, providing students a visual and kinetic experience with math, thereby deepening conceptual understanding.
The following are some math art projects and lesson ideas that you could use in your math centers, as whole group lessons, to differentiate lessons, as homework, after school, or with your child at home.
Ages: Pre-K - kindergarten
Standards:
Look for and make use of structure [CCSS.MATH.PRACTICE.MP7]
Look for and express regularity in repeated reasoning [CCSS.MATH.PRACTICE.MP8]
Explore patterns with younger students. Start by showing examples of patterns, such as AB, ABC, or AABB. Ask students to create their own similar patterns.

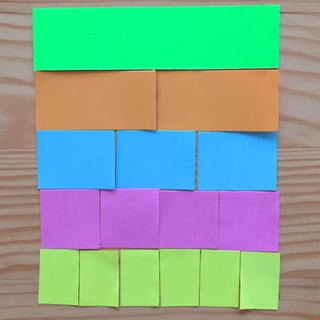
Design a mosaic tile. This could be a pattern or an image. You can also elevate the task by giving it more context such as asking students to play architect and design a floor plan for a dream house, or be an interior decorator by designing a backsplash or floor tile design. This is also reminiscent of graphic designers who design small icons pixel by pixel. You can further differentiate this by changing the number of colors a student can use or the area/dimensions of the mosaic.
From here you can explore a variety of math topics:
Ages: 3rd - 4th graders
Standards:
Understand concepts of area and relate area to multiplication and to addition. [CCSS.MATH.CONTENT.3.MD.C.6]
[CCSS.MATH.CONTENT.3.MD.C.7]
Solve problems involving measurement and conversion of measurement.
[CCSS.MATH.CONTENT.4.MD.A.3]
Learning Objectives: Identify the perimeter and area of each color.
Because these are atypical shapes and designs unique to each student, this will really push students to confront and solidify their understanding of perimeter (as the outer edge) and area (as the space inside).
Ages:3rd graders
Standards: Develop understanding of fractions as numbers. [CCSS.MATH.CONTENT.3.NF.A.1]
Learning Objective: Identify the fractional amount of each color in the overall design.
Students can inspect the proportions of each color used in their design. This will push students’ understanding of parts and wholes.
Ages:4th - 5th graders
Standards:
Develop understanding of fractions as numbers. [CCSS.MATH.CONTENT.3.NF.A.3]
Build fractions from unit fractions [CCSS.MATH.CONTENT.4.NF.B.3, CCSS.MATH.CONTENT.4.NF.B.4]
Use equivalent fractions as a strategy to add and subtract fractions. CCSS.MATH.CONTENT.5.NF.A.1]
Apply and extend previous understanding of multiplication and division. [CCSS.MATH.CONTENT.5.NF.B.4, CCSS.MATH.CONTENT.5.NF.B.6, CCSS.MATH.CONTENT.5.NF.B.7.B]
Learning Objectives: Adding, multiplying, and dividing fractions
Starting with long strips of paper, ask students to subdivide the strip into fractional parts to create their own fraction tiles. Let each color be its own unit fraction. Use these more complex tiles to create a mosaic design.
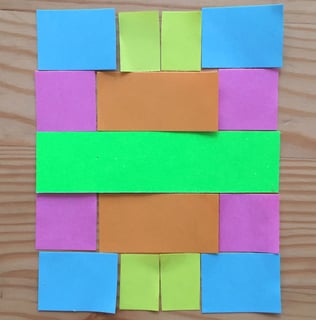
Ages: 6th - 7th graders
Standards: Summarize numerical data sets in relation to their context. [CCSS.MATH.CONTENT.6.SP.B.5]
Investigate chance processes and develop, use, and evaluate probability models. [CCSS.MATH.CONTENT.7.SP.C.6]
Turn your mosaic into a game board. Create a border around the edge. (You could use snap cubes, tape, more paper, pipe cleaners, etc.) Add a small token into the mix - a top or a coin to spin, a die or chip to toss. The “game” is that you’re going to toss or spin this token onto this design and see where it’ll land.
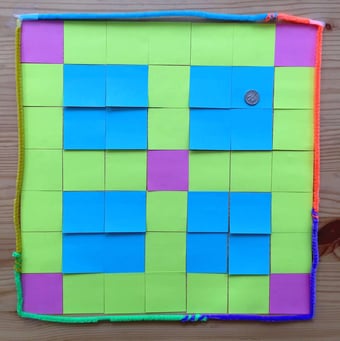 Students can then test their calculations by conducting trials and finding the experimental probability of how often the token lands on each color. This is a great opportunity for students to observe and collect actual data.
Students can then test their calculations by conducting trials and finding the experimental probability of how often the token lands on each color. This is a great opportunity for students to observe and collect actual data.
You can see that you could blend a lot of the above activities together to create hybrid activities where even more interesting questions will emerge. For example, the game board students create in the mosaic tiles probability activity could be used as a starting point for the K-12 Game-a-thon challenge.
This allows students to see the interconnectedness between math topics - how do fractions relate to area and perimeter, and then to probability? The art of tiling can facilitate discussion and motivate questions across multiple math topics, and can reach students of all levels. And since the designs are made by the student, they will be more invested in the math. It beats answering textbook word problems about Bob’s garden planter any day!
What else can you do with paper tiles and art in math? Read how one student created a visually engaging board game for the K-12 Game-a-thon challenge.
The art of tiling is an ancient one (as seen in Islamic tilings starting in 1200) and has been utilized by many prominent artists, spans many cultures, and even has scientific implications.

Nina Wu is an Educational Game Designer at MIND Research Institute. She enjoys finding the math in her daily hobbies, including dance and art.
Comment